quicksasa.blogg.se - Gauss jordan elimination example

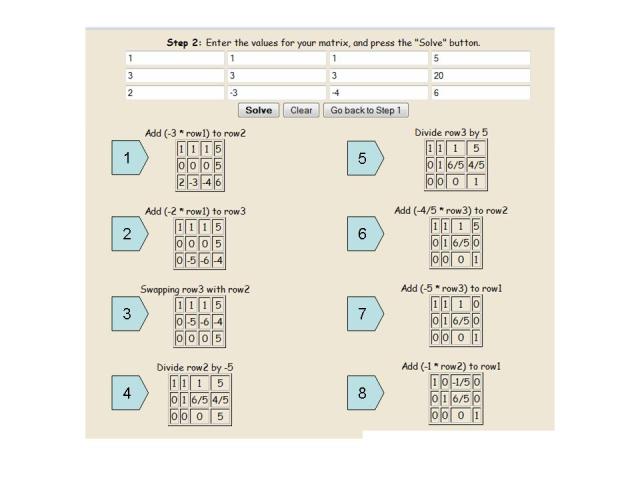
įrom the matrix in slide 10, the new matrix becomes: 1 – 3/4 7/4 0 1 3 ( 4) R2 Remember that when you change a pivot number to a 1, you use the second elementary row operation Multiply an equation by a nonzero.The pivot value is now 1/4 and the pivot row is the 0 1/4 3/4 row (i.e.Since all the values below the pivot value of 1 are now zeros, the pivot value moves down the diagonal.Thus the new matrix will be the following:įrom the matrix in slide 6, the new matrix becomes: 1 – 3/4 7/4 0 1/4 3/4 R2 + (– 3) R1 Ĭhange the pivot number 1 – 3/4 7/4 0 1/4 3/4.Now since R2 is at the beginning of the statement R2 + (– 3) R1, replace row 2 with the 0 1/4 3/4 values.The row of values 0 1/4 3/4 comes from adding the corresponding values in the two rows above, hence the addition symbol in the notation R2 + (– 3) R1.(– 3) R1 means multiply (– 3) to the values in row 1. On a scratch piece of paper, do the following row operation: R2 + (– 3) R1 R2 (– 3 ) R1 3 – 2 6 – 3 9/4 –21/4 0 1/4 3/4

In this case we want to change the 3 (in the second row, first column) to a 0, so we take the second row and add it to ( – 3) times the values in the pivot row.

change the sign of the number) that you want to change to a 0.

Do this by multiplying the pivot row by the opposite number (i.e.

Ĭhange any values above and or below the pivot value to a 0.

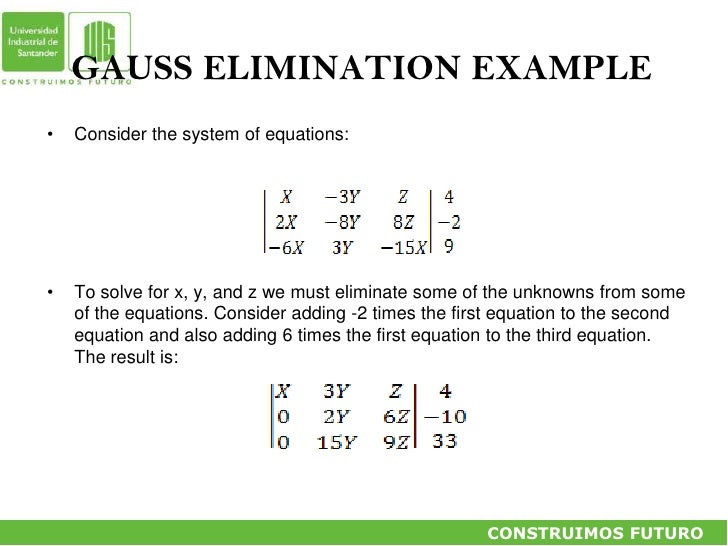
The notation (1/4)R1 means to multiply all the values in row 1, as signified by the R1, by the value of (1/4), which is the reciprocal of 4.įrom the matrix in slide 4, the new matrix becomes: 1 – 3/4 7/4 3 – 2 6 (1/4) R1 Remember that when you change a pivot number to a 1, you use the second elementary row operation Multiply an equation by a nonzero value.Ĭhange the pivot number to a 1 by multiplying the pivot number, and all the other numbers in the pivot row,by the reciprocal of the circled number. Recall that the row with the pivot number (circled number) is called the pivot row.Pivot Number and Pivot Row 4 – 3 7 3 – 2 6 The system of linear equations 4x – 3y = 7 3x – 2y = 6 Ĭonvert to a matrix of coefficients 4x – 3y = 7 3x – 2y = 6 4 – 3 7 3 – 2 6 Now circle the pivot number. Gauss – Jordan Elimination Method: Example 1 Solve the following system of linear equations using the Gauss-Jordan elimination method